Mom and Me Math Activities: 4.OA.C.5 Algebra Growth Patterns

Welcome to Mom and Me Math Friday!

In this lesson, the children started with a basic design unit and then built sequential steps of their pattern, showing how it grew systematically. Here you can see Nathan and his mother working on her "Rose Garden" design.

I asked each child, "What is the rule? How is your pattern growing? Do you need to revise anything so that each step follows your rule?"

"Mom's design is very fancy! Can you help her clarify her thinking and finish building the pattern so it matches her rule?" I asked Nathan.

On Monday morning, Nathan was extremely proud to see Mom's design displayed up on our wall!! Great job, Mom!! We are all proud of you.

Diane made "bumblebees." Each bee needed 8 blocks. "My rule is multiply by 8," she smiled. "Because 1 x 8 is 8, and 2 x 8 is 16, and 3 x 8 is 24, so that's how I know."

The children made t-charts to record their growth patterns. On the input side (x), I told them to write the name and number of times they built their design. On the output side (y), I told them to write the "number of blocks" necessary to build that many of their design each time.

Ana called her design "Fire Turtles."

"I'm counting by nines," she proudly announced. "So my rule is multiply by nine."

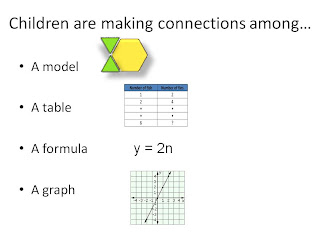
I showed the parents how to help their children make important algebra connections by building a model, creating a table, finding their rule or formula, and graphing it.

How many fins does one fish have?
How many fins do two fish have?
How many fins do 10 fish have?

How can I find out how many fins 100 fish have?

Kurt made robots. Each robot needed 9 blocks, so his formula is y = 9n.

I still need to teach the children the importance of accuracy and being precise, but we are making progress! Kurt made his robots and saw the rule of multiplying by 9, but counted by tens when he wrote this on his t-chart.

Math is fun when it is relevant to the lives of the children. How exciting to make a design that uses the colors of your heritage! Parents and children enjoyed sharing their designs with the class.

I often create PowerPoints for the children. This helps them stay focused on the directions, and allows them to reflect on previous learning. I usually show them photographs of what happened on the previous day, and allow them to build on those ideas--modifying, clarifying, and constructing new knowledge as we go.

Fatima sparkles as she shares her "Dancing Ducks" and her rule of multiply by 8.

Saul saw that his formula was y = 7n, because each time he needed 7 more blocks to make his rockets.

The following day, we made ordered pairs and graphed our designs.

I gave the students a communication guide and showed them a rubric of how I would grade their work.

Anthony needed 8 blocks to create each of his "Droids." Below is the communication guide he used to write his reflection.

The children had to use pictures, numbers, and words to prove and clearly show their thinking. Everyone felt successful, had a chance to review their time tables, and made important connections with algebra.

Other algebra lessons we did...

This time we did the Marilyn Burn's activity called Banquet Tables.

Anthony knows that the rule is "multiply the table number (n) by 2 and then add 2."

Tommy and Diane said that if you had 1,000 tables, then you would need 2,002 chairs.

After discussing what operations we did each time, the children were able to see that the formula was y = 2n + 2, or multiply two times the table number (n) and add 2 for the two end seats.

Success!!

Here the growth patterns are introducing the concept of multiplying double-digit numbers. In this case, the children are determining...
"What is 4 x 14?

Below are some of our other growth pattern designs: