4.G.A.3 Symmetry

4.G.A.3 Recognize a line of symmetry for a two-dimensional figure such that the figure can be folded along the line into matching parts. Identify line-symmetric figures and draw lines of symmetry.

We had a great time folding paper and using pattern blocks to create beautiful symmetrical designs...as well as reading "Snowflake" Bentley in our Treasures reading book.

We do not have snow in Southern California, so the children are fascinated by the snowflake designs they can create. (We do know they have a pattern of 6 points, but that's about it.)

Esmeralda created this design with two lines of symmetry. The weather dropped down to the high 60's and I like the classroom window open for fresh air. Hence, the Eskimo look.  :)

If you have a mirror, it's a great opportunity for investigation to actually see the lines of symmetry in the various patterns.

Cristal's Girl design has one line of symmetry.

Here are some other 
one-line-of-symmetry designs.

Can you see Patricio's Angry Bird face in his design?













Esmeralda's Dancing Girl is kicking one leg up a little too high, but you get the symmetrical idea.

 We also cut designs out of paper. Messy, but worth the adventure.

 Silly Angel!!

Great job, Angel R!

Hooray for Mrs. Fuerte!! Two lines of symmetry and very beautiful.

Patricio's 
one-line-of-symmetry tower.

So many creative designs!

Great job, ladies.   :)
Hum...these show rotational symmetry.

Rotational Symmetry Examples

More Rotational Symmetry

A polygon has rotational symmetry if it maps back onto itself after turning or rotating it less than 360 degrees. Trace the figure on paper, hold it in the middle, and spin it around this center to see if it matches again before returning to the original position.









Fatima can spin her design 180 degrees, so its order of rotational symmetry is two. It matches twice as it rotates and 2 x 180 = 360.

Galilea created a 5-pointed star. Its order of rotational symmetry is 5. Its angle of rotation is 72 degrees, because 5 x 72 = 360.










Kimberly made ones that rotated 4 and 8 times.

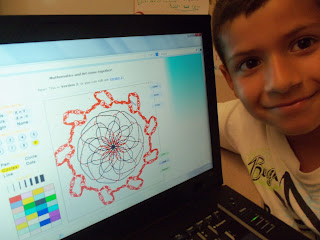
The computer exploration is unlimited fun. What a great way to celebrate the life of "Snowflake" Bentley!   :)

Eligio, I'm so impressed with your spectacular drawing of Mario!!  Great reflection across the x-axis!

Symmetry Artist website

Fabulous, Angel!

All of these are beautiful!!