 4.NBT.5 Multiply a whole number of
up to four digits by a one-digit whole number, and multiply two two-digit
numbers, using strategies based on place value and the properties of
operations. Illustrate and explain the calculation by using equations,
rectangular arrays, and/or area models.
4.NBT.5 Multiply a whole number of
up to four digits by a one-digit whole number, and multiply two two-digit
numbers, using strategies based on place value and the properties of
operations. Illustrate and explain the calculation by using equations,
rectangular arrays, and/or area models.
PARCC Buses, Vans, and Cars Then click on Elementary School Tasks to find this activity.
 How many children and teachers were going on the fieldtrip?
How many children and teachers were going on the fieldtrip?
Our assumptions did not influence the final answers, but the discussion did engage the class! Even our teacher, Mr. Brown, was eager to find the solution.
First, we had 4 minutes of silent reading and independent work time, before sharing answers in our small table groups.
We all knew algorithms to correctly solve the problems, but how could we justify our answers?
Mrs. Penniman said we had to use mathematical pictures, numbers, and words to prove our solutions. She also said we could use the distributive property to find our answers. But how can we do that?

Soon we started getting a game plan.
"If 3 of the solutions are correct and 2 are incorrect, which ones are the right answers?" we wondered.
"Okay, there are 72 people going on the fieldtrip," we decided. "But none of the possible answers match this!"
Some began to wonder, "So are all of the answers wrong?"

It took several minutes for some groups to decide that there could be empty seats on the bus, in the vans, or in the cars.
Whew! We're making progress!
Answers: Why or Why Not? Do They Work?
Answer #1: 1 bus and 4 vans
1 bus = 20 seats
4 vans = 16 x 4 = 64 seats.
72 people and 84 seats.
"It doesn't work because 84 is too big of an number to fit 72. There is 12 more seats left all together."
Again on #2, the student thought that 103 seats was too many seats for only 72 people, "so it doesn't work. There is 31 seats that are available."
Answer #3: "72 people and 66 seats. It completely doesn't work, because there is only 66 seats. Many kids will be left out."
When the number of available seats was less than the 72 students, the children were able to understand why this was not a viable answer. "You can't leave kids at school, it wouldn't be fair. Everybody gets to go on the school trip--unless they're absent."
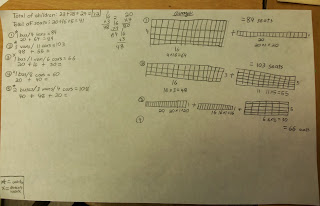
Correct solutions! And beautiful arrays... but now can you write sentences that justify your answers?

Although the class knew how to multiply with double-digit numbers, most preferred to show their work using repeated addition.
However, counting on fingers and writing long columns of numbers can make for careless mistakes.

I praised this young man for his simple math pictures. (Notice his 3 rectangles with 16 written in each, representing the vans.) These simple pictures do the job and save valuable problem-solving time.

 Finally, it was time to publish our answers and display them on posters for everyone to admire.
Finally, it was time to publish our answers and display them on posters for everyone to admire.This takes the most planning of all. How can we convey our answers in a way that makes sense to others?
Stay tuned for our finished products!
















awesome!
ReplyDeleteI'm delighted that you enjoyed participating with us today at UCLA and I'm eager to hear how it works for you with your own students.
Delete