Enlarging Objects
After reading the story, Clifford's Tricks, by Norman Bridwell, I showed my students how to determine Clifford's size relative to that of Bruno, the real world dog.
On the first page of the book, Bruno measures one inch from the bottom of his foot to the top of his shoulder. Two pages later, Clifford can also be measured in this same manner, from the bottom of his paw to the top of his shoulder. This measurement for Clifford is four inches, so I told the children that we have a scale factor of 4 to 1. This means that Clifford's dog bone, dog toys, doghouse, etc., are all 4 times larger than Bruno's toys or doghouse. 
This inspired the class to investigate.
The children brought dog items from home, including rubber chew toys, collars, dog bones, and paw prints.
I asked my third graders if they thought Clifford's doghouse could fit in our classroom and their eyes widened. "Wow! It would be enormous!" they decided.
Sammy is holding Gustavo J.'s artwork. Gustavo traced around his small plastic toy of Iago from the Disney movie Aladdin.
Gustavo "Goose" drew his Gumby eraser and then both boys enlarged their drawings onto the larger grid paper.
Alejandra drew a freehand picture of the Bad Kitty character from Nick Bruel's book series. The trick is to not look at the whole picture; rather, just look at one individual square and draw that shape in the corresponding square on your larger paper.
My third graders did so well with this project, that I decided to try it again this year with my fourth grade class.
Kimberly traced around her eraser on the small graph paper and then enlarged it to make it a suitable size for Clifford. The children soon realized that the new eraser was 4 times wider and 4 times longer--so the new area was actually 16 times larger! This surprised many of them.
Shrinking Objects
Thumbelina by Hans Christian Andersen is always a delightful story to use for this activity. In doing a little research, the children and I discovered that a field mouse can grow to a length of 90 mm from the tip of its nose to the start of its tail. In the illustrations, Thumbelina appears to be about the size of a field mouse, so she could be about 3 inches tall. This also seems reasonable to us since she can sit in the palm of your hand.
So...in the real world, Thumbelina might be 5'6", and if so, then this would be a scale of 22 to 1. She's pretty tiny!
Another great book to use for shrinking objects is Indian in the Cupboard, by Lynne Reid Banks. Here the Indian is also about the size of Thumbelina.
Cesar traced his bookmark on the small grid paper and then just traced around his hand to enlarge it. The concept of looking at each square and only drawing what appeared inside that one square was beyond many of my students. Maybe next time I'll have them turn the original picture upside down so their mind does not interfere with what they think it should look like.
Jessi is enlarging his muscle man.
Jason enlarges SpongeBob SquarePants.
Esmeralda has fun enlarging her house key. She and Jason really worked hard at making their shapes appear to be similar. I am very proud of their efforts.
Excellent work!
I showed the children how to trace around an object and then either enlarge or shrink it 4 times. "When you enlarge, you multiply by 4. When you shrink, you multiply by 1/4," I told the class.
Such a terrific end-of-the-school-year activity!
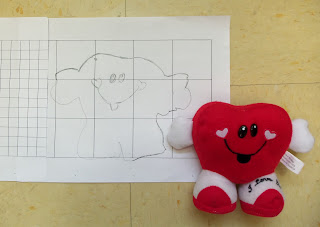
Angie has fun shrinking the valentine toy.
Kimberly holds her Hello Kitty plastic car that was the source of her inspiration.
Patricio has fun shrinking his enormous Spider-man picture.
Alexis and Rigoberto enlarge their own creations. The creativity in this activity is endless and makes the children feel more grown up, since they are doing a 5th grade CORE math standard. :)
Great fun!
When you have a scale factor of 4, what happens to the area?
